Contribution to Book
Mathematical derivations of inscribed & circumscribed radii for three externally touching circles (Geometry of Circles by HCR)
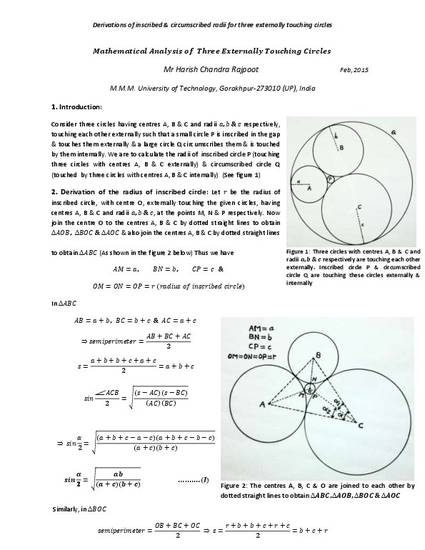
Mathematical Analysis of Three Externally Touching Circles
(2015)
Abstract
All the articles, related to three externally touching circles, have been derived by using simple geometry & trigonometry to calculate inscribed & circumscribed radii. All the articles (formula) are very practical & simple to apply in case studies & practical applications of three externally touching circles in 2-D Geometry. Although these results are also valid in case of three spheres touching one another externally in 3-D geometry. These formula are also used for calculating any of three radii if rest two are known & the dimensions of the rectangle enclosing thee externally touching circles. Here is also the derivation of a general formula for computing the length of common chord , area of intersection and angle of intersection of two intersecting circles.
Keywords
- derivations of inscribed & circumscribed radii for three externally touching circles
Disciplines
Publication Date
Winter February 15, 2015
Publisher Statement
outstanding
Citation Information
Harish Chandra Rajpoot. "Mathematical derivations of inscribed & circumscribed radii for three externally touching circles (Geometry of Circles by HCR)" GorakhpurMathematical Analysis of Three Externally Touching Circles (2015) Available at: http://works.bepress.com/harishchandrarajpoot_hcrajpoot/31/
